even + even is equal to|Rules for Even and Odd Numbers (worksheets, : Baguio Even numbers are those numbers that can be divided into two equal groups or pairs and are exactly divisible by 2. For example, 2, 4, 6, 8, 10, and so on are even numbers. Watch Milf Shopping porn videos for free, here on Pornhub.com. Discover the growing collection of high quality Most Relevant XXX movies and clips. No other sex tube is more popular and features more Milf Shopping scenes than Pornhub! Browse through our impressive selection of porn videos in HD quality on any device you own.
even + even is equal to,Even Numbers. Any integer that can be divided exactly by 2 is an even number. The last digit is 0, 2, 4, 6 or 8. Example: −24, 0, 6 and 38 are all even numbers.When one number is even and the other odd, the product of the numbers is even. (even × odd = even or odd × even = even) Show Video Lesson. Using the properties of odd and even numbers - Division. Use multiplication with pairs of .
Even numbers are those numbers that can be divided into two equal groups or pairs and are exactly divisible by 2. For example, 2, 4, 6, 8, 10, and so on are even numbers.
Yes, the difference between two even numbers is always even. For example, 86 and 72 are two even numbers, where the difference 86 – 72 = 14, is even. Difference between odd and even .

An even number can be divided into two equal groups. On the other hand, an odd number cannot be divided into two equal groups. Learn more about even and odd numbers with concepts, definitions, properties, and examples.

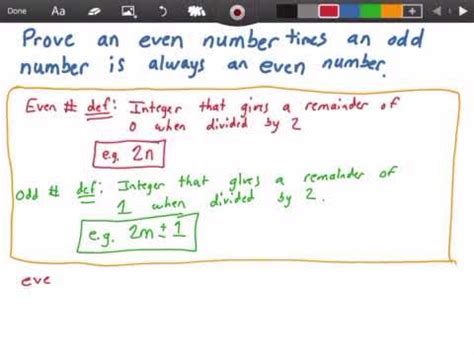
Definition. An even number has parity 0 0 because the remainder upon division by 2 2 is 0 0, while an odd number has parity 1 1 because the remainder upon division by 2 2 is 1 1. For example, 0,2,4,10,-6 0,2,4,10,−6 are all even .Property of multiplication. If you multiply an even number by an even number, the product will always be an even number. For example, 4 × 2 = 8.4 × 2 = 8. If you multiply an even number by an odd number, the product will always be an .even + even is equal to The 3 main properties are: Property of Addition. The summation of 2 even numbers is an even number (Even + Even = Even). For example, 2 + 8 = 10. The summation of an even and an odd number gives an odd number .An even number is an integer that can be divided by 2 such that there is a remainder of 0. In other words, any integer that is a multiple of 2 is even. Generally, even numbers are numbers that .Even Numbers are integers that are exactly divisible by 2, whereas an odd number cannot be exactly divided by 2. The examples of even numbers are 2, 6, 10, 20, 50, etc. The concept of even number has been covered in this lesson . Break-Even Point (BEP) Definition . The break-even point is the volume of activity at which a company's total revenue equals the sum of all variable and fixed costs. The activity can be expressed in units or in dollar .
Examples of Solving the Sum of Consecutive Even Integers . To get a good grasp of the procedure, let’s begin by solving a simple problem. Example 1: Find the two consecutive even numbers whose sum is [latex]66[/latex].. Let .
The sum of first consecutive even numbers is equal to n(n+1). Learn to find the sum of even numbers using Arithmetic Progression and sum of natural numbers formulas at BYJU’S. . Find the sum of even numbers from 1 to 200? Solution: We know that, from 1 to 200, there are 100 even numbers. Thus, n =100. By the formula of the sum of even . The break-even point for businesses occurs when revenue and expenses are equal. Alternatively, it is the amount of revenue that a business needs to earn in order to cover both its fixed and variable costs. Before reaching its break-even point, the business will operate at a loss since its revenue doesn’t cover all of its costs.
We would like to show you a description here but the site won’t allow us.Rules for Even and Odd Numbers (worksheets, The sum of two even functions will also be even. The composition of two odd functions will also be odd. Let’s say f(x) is odd, so f(-x) is equal to -f(x). Taking the absolute value of this function returns f(x) back. This means that the function is even. Example 2The break-even point is a crucial concept in business and finance. It refers to the point at which total revenues equal total costs, resulting in neither a profit nor a loss. Understanding the break-even point is vital for businesses as it helps them determine the minimum level of sales necessary to cover all their expensesAs for the constant term, I must add that it can also be expressed as [latex] – 1 = – 1{\color{blue}{x^0}}[/latex] which has an even power of zero. This characteristic of a function containing only even powers can likely result in an even function. However, we must show it algebraically. So here it goes. Sample Spaces and Events. Rolling an ordinary six-sided die is a familiar example of a random experiment, an action for which all possible outcomes can be listed, but for which the actual outcome on any given trial of the experiment cannot be predicted with certainty.In such a situation we wish to assign to each outcome, such as rolling a two, a number, called the . Let $2m$ be an arbitrary even number. Add $1$. Then $2m+1$ is odd. Assume $2m+(2n+1)$ is odd for all odd numbers less than or equal to $2n+1$. Consider the next odd number. We have $$2m+(2(n+1)+1)=2(m+n+1)+1$$ is odd by definition (as it is one away from a multiple of two). But $2m$ was arbitrarily even. $\square$ Given a range [L, R], the task is to count the numbers which have even number of odd digits and odd number of even digits. For example, 8 has 1 even digit and 0 odd digit - Satisfies the condition since 1 is odd and 0 is even.545 has 1 even digit and 2 odd digits - Satisfies the condition since 1 is odd and 2 is even.4834 has 3 even digits and 1 odBreak-even point is the point where revenues equal the total of all expenses including the cost of goods sold. True. Right! If revenues minus all expenses (fixed and variable, and including cost of goods sold) equals zero, you are at the break-even point. False. Wrong.To calculate the probability of an event A when all outcomes in the sample space are equally likely, count the number of outcomes for event \(\text{A}\) and divide by the total number of outcomes in the sample space. For example, if you toss a fair dime and a fair nickel, the sample space is \(\{\text{HH, TH, HT,TT}\}\) where \(\text{T .even + even is equal to Rules for Even and Odd Numbers (worksheets, Therefore, the break-even point for each unit equals 1,400. This means that the revenue from 1,400 guest nights is the point at which all variable costs and fixed costs can be paid, without any money left over. In order to break even for the year, B&B needs to have a 40% occupancy rate (1,400 sold / 3,550 total rentable nights per year). .
Note: The purpose of brainstorming in writing proof is for us to understand what the theorem is trying to convey; and gather enough information to connect the dots, which will be used to bridge the hypothesis and the conclusion. At the back of our head, we should know what an even number looks like. The general form of an even number is shown below. Properties of Even Signals. The properties of the even signals are given as follows −. The even signals are symmetrical about the vertical axis. The value of an even signal at time (t) is same as at time (-t). The even signal is identical with its reflection about the origin. Area under the even signal is two time of its one side area. Odd SignalHow can you find the sum of consecutive integers using linear equations? Watch this video from Khan Academy and learn how to set up and solve equations word problems involving consecutive numbers. Khan Academy is a free online learning platform .
even + even is equal to|Rules for Even and Odd Numbers (worksheets,
PH0 · What are Even Numbers? Definition
PH1 · Rules for Even and Odd Numbers (worksheets,
PH2 · Even numbers
PH3 · Even and Odd Numbers
PH4 · Even Numbers and Odd Numbers – Properties, Examples
PH5 · Even Numbers
PH6 · Difference between Odd and Even Numbers